Have you ever tried to navigate a crowded amusement park, dodging excited children and maneuvering around massive roller coasters? Or perhaps you’ve found yourself attempting to throw a basketball through a hoop, aiming for that perfect arc to ensure a satisfying swish? These seemingly simple tasks, common to our everyday lives, are actually intricate examples of motion in two dimensions. This fascinating aspect of physics, often explored in Mechanics homework, delves into the world of movement across a plane rather than a single line, offering insights into everything from the trajectory of a rocket to the path of a tossed frisbee.
Image: www.studocu.com
Understanding motion in two dimensions is crucial not only for tackling those challenging Mech HW-21 assignments but also for comprehending the world around us. It lays the foundation for understanding forces, work, energy, and momentum, all vital concepts in mechanics and beyond. Whether you’re a budding scientist, an avid athlete, or simply someone intrigued by the way things move, this exploration will shed light on the fascinating principles that govern the movements we see and experience daily.
Defining the Foundation: Key Concepts in Two-Dimensional Motion
Before diving into the specifics, let’s lay the groundwork by understanding some cornerstone concepts. Motion in two dimensions involves describing the movement of an object across a plane, often using a coordinate system. This system helps us track both the horizontal and vertical components of the object’s position, velocity, and acceleration.
Here’s a breakdown of the key terms:
- Position: Defined by its (x,y) coordinates within the chosen coordinate system. Imagine it as the object’s “location” on a map.
- Displacement: The change in position, the difference between the initial and final positions of the object. This is a vector quantity, meaning it possesses both magnitude and direction.
- Velocity: It’s the rate of change of position over time. A vector, it describes both the object’s speed and the direction of its motion.
- Acceleration: The rate of change of velocity over time. Also a vector, it indicates how quickly the object’s speed and/or direction is changing.
The Power of Vectors: Unveiling the Direction of Motion
Vectors are essential tools in analyzing motion in two dimensions. They represent quantities that have both magnitude and direction.Think of them as arrows pointing in specific directions. Let’s see how they bring clarity into our understanding:
1. Graphical Representation: Vectors are visually depicted as arrows, where the length of the arrow represents the magnitude of the quantity, and the direction of the arrow points towards the direction of the quantity. For example, a longer arrow represents a greater speed.
2. Adding and Subtracting Vectors: Just like we can add numbers, we can combine vectors. The resultant vector is simply the sum of the individual vectors. To subtract two vectors, we can simply add the negative of the second vector to the first vector. This is extremely helpful in situations involving multiple forces acting on an object.
3. Resolving Vectors: Imagine a ball rolling diagonally across a table. Its motion can be broken down into its horizontal and vertical components using vector resolution. This is achieved by finding the projection of the vector onto the horizontal and vertical axes.
Unraveling the Movement: Equations of Motion
Now, let’s explore the mathematical formulas that govern motion in two dimensions. These equations are crucial for predicting the path of an object and understanding its motion through time.
1. Constant Velocity Motion: In scenarios where the object’s velocity remains constant, we can use simple equations to determine position, displacement, and velocity. For example, the position of the object at any time can be expressed by the equation:
Position = Initial Position + (Velocity * Time)
2. Uniformly Accelerated Motion: When the object’s velocity changes at a constant rate, we use equations of uniformly accelerated motion. These equations encompass gravity, a crucial force affecting motion on Earth. An example of such equations is:
Final Velocity2 = Initial Velocity2 + 2 * Acceleration * Displacement
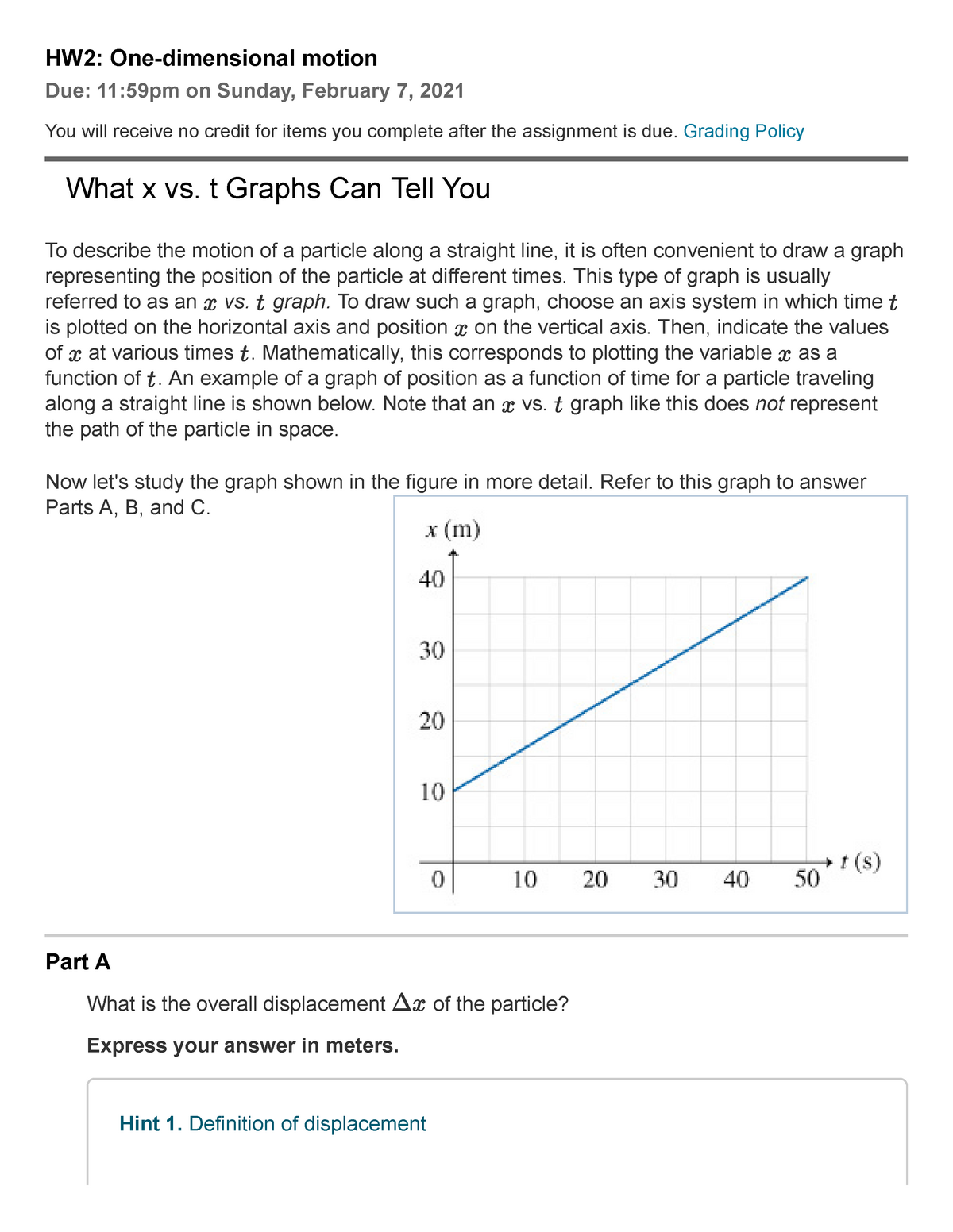
Image: www.studocu.com
Real-World Applications: Where Motion in Two Dimensions Reigns
The principles of motion in two dimensions aren’t confined to the realm of textbooks. They manifest themselves in countless real-world scenarios, influencing our daily lives and the technological marvels that surround us. Here are some notable applications:
1. Sports: Think of a baseball player hitting a home run. The ball’s trajectory, influenced by gravity, air resistance, and the initial force of the bat, accurately depicts motion in two dimensions. This same principle applies to basketball shots, soccer kicks, and even the graceful flight of a badminton shuttlecock. Understanding two-dimensional motion helps athletes improve their performance.
2. Navigation: Airplanes, ships, and even spacecraft rely on the principles of motion in two dimensions for navigation. Pilots and navigators use these concepts to chart courses, calculate flight paths, and ensure safe and efficient travel.
3. Projectile Motion: From the trajectory of a bullet fired from a gun to the arc of a gymnast performing a somersault, projectile motion is a prime example of two-dimensional movement. Understanding the factors that influence the trajectory, such as initial velocity, launch angle, and air resistance, is essential for predicting the path and landing location of the projectile.
Expanding the Horizon: Delving Into Advanced Topics
While we’ve covered the fundamental aspects of motion in two dimensions, the topic extends far beyond the basics. As you delve deeper into Mechanics, you’ll encounter advanced concepts like:
1. Rotation: While we’ve focused on translational motion (movement from one point to another), rotating objects also exhibit motion in two dimensions. This involves concepts like angular velocity, angular acceleration, and torque. Understanding these concepts is vital for analyzing the motion of wheels, gears, and other rotating systems.
2. Relativity: In the realm of Einstein’s theory of relativity, motion in two dimensions takes on a whole new dimension. Space and time are intertwined, and the speed of light plays a critical role in understanding motion at extreme speeds. This concept is relevant in understanding the movements of celestial bodies and the nature of our universe.
3. Fluid Dynamics: The study of fluids (liquids and gases) involves intricate patterns of motion in two dimensions. Understanding fluid flow, turbulence, and buoyancy is fundamental in areas like aerodynamics, hydraulics, and meteorology.
Motion In Two Dimensions Mech Hw-21
https://youtube.com/watch?v=mAQ9_nLGTkc
Conclusion: Unleashing the Power of Understanding Motion
As we conclude our exploration of motion in two dimensions, it’s clear that this seemingly simple topic holds the key to understanding the complexities of our world. From the graceful flight of a bird to the intricate workings of machinery, motion spans countless facets of our experience. By delving into the fundamental concepts, equations, and real-world applications of two-dimensional motion, you’ll equip yourself with valuable knowledge and a deeper appreciation for the beauty and elegance of the physical world. So, explore further, delve into the intricacies of Mech HW-21, and unleash the power of understanding motion in all its dimensions. Who knows what fascinating discoveries await you?






