Remember those frustrating geometry problems where you were given a diagram full of angles and asked to find their measures? It’s like deciphering an ancient code, but thankfully, there’s a key to unlocking the mystery! This key lies in understanding the relationships between different types of angles, which allows you to make deductions and solve for the unknown. In this blog post, we’ll explore these relationships and provide a step-by-step guide on using them to find angle measures, ensuring that you’ll never be stumped by another geometric puzzle again.
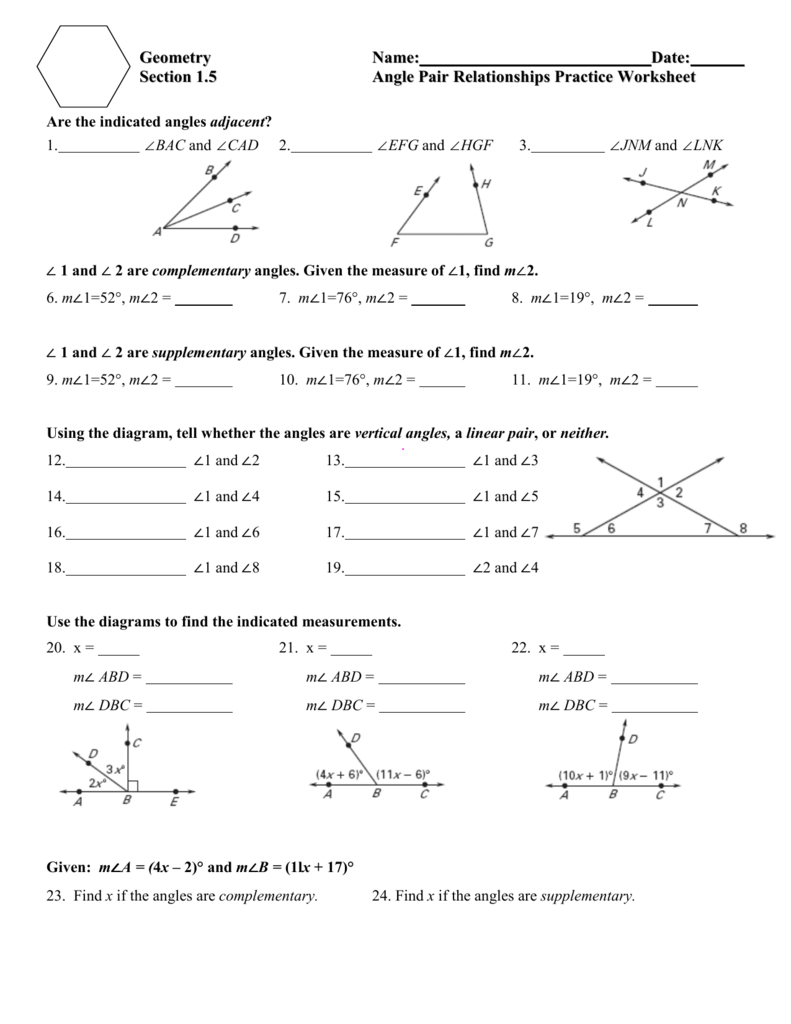
Image: answerlibrosa.z13.web.core.windows.net
Whether you’re a student struggling with geometry or a curious learner wanting to refresh your understanding of these fundamental concepts, we’ve got you covered. We’ll delve into the world of angles, uncovering their secrets and revealing the connections that exist between them. Get ready to unlock the power of angle relationships and master this vital skill in the fascinating field of geometry!
A Deeper Dive into Angle Relationships
Angles are a fundamental concept in geometry, defining the space between two intersecting lines or rays. Understanding the relationships between different angles is crucial for solving a wide range of geometrical problems. There are several key angle relationships that you should be familiar with:
1. Complementary Angles: Two angles that add up to 90 degrees are called complementary angles. Imagine a right angle, split into two pieces – that’s a complementary pair! This relationship is essential for working with triangles and other shapes that contain right angles.
2. Supplementary Angles: Two angles that add up to 180 degrees are called supplementary angles. Think of a straight line, divided into two sections, each forming a supplementary angle. This relationship is particularly useful when dealing with angles on a straight line or when dealing with angles inside a triangle.
3. Vertical Angles: When two lines intersect, they form four angles. The angles opposite each other are called vertical angles, and they are always equal. Imagine an ‘X’ formed by the intersecting lines; the angles at the top and bottom of the ‘X’ are vertical angles, as are the angles on the left and right.
4. Adjacent Angles: Adjacent angles share a common vertex and a common side but don’t overlap. Think of two angles forming a corner of a room; they share the corner (vertex) and a wall (common side). Understanding adjacent angles helps you solve problems involving angles formed by parallel lines.
5. Corresponding Angles: Corresponding angles occur when two parallel lines are intersected by a transversal (a line that cuts across the parallel lines). These angles occupy the same relative position at each intersection point, and they are always equal.
6. Alternate Interior Angles: When two parallel lines are intersected by a transversal, the angles inside the parallel lines and on opposite sides of the transversal are called alternate interior angles. They are also always equal.
7. Alternate Exterior Angles: Similar to alternate interior angles, alternate exterior angles are formed when two parallel lines are intersected by a transversal, but they are located outside the parallel lines and on opposite sides of the transversal. Like their interior counterparts, they are also always equal.
Mastering the Art of Finding Angle Measures: A Step-by-Step Guide
Now that you have a solid grasp of the major angle relationships, let’s dive into the practical applications of these concepts. Here’s a step-by-step guide to finding angle measures using angle relationships:
Step 1: Identify the Known Angles: Start by carefully examining the given diagram. Identify all the angles that are labeled with their measures. These known angles will serve as your starting point.
Step 2: Identify the Unknown Angles: Mark the angles for which you need to find the measure. These will be your targets.
Step 3: Find the Relationship: Determine the relationship between the unknown angles and the known angles. Are they complementary, supplementary, vertical, or adjacent?
Step 4: Apply the Relationship: Use the appropriate equation or rule based on the identified relationship to solve for the unknown angles. For example, if two angles are supplementary, add them together to equal 180 degrees.
Step 5: Solve for the Unknown Angle: Use basic algebraic skills (like subtracting or dividing) to isolate the unknown angle in your equation.
Step 6: Repeat for All Unknown Angles: Continue applying the same process for each of the unknown angles in the diagram, working your way through each angle until all have been solved.
Example: Applying Angle Relationships to a Problem
Imagine a diagram with two parallel lines intersected by a transversal. Let’s say one angle is labelled 75 degrees, and you need to find the measure of another angle formed by the transversal. Following the steps above:
1. Known Angle: 75 degrees.
2. Unknown Angle: The angle we need to find.
3. Relationship: The unknown angle and the given angle are corresponding angles (because they’re in the same relative position on both lines).
4. Apply Relationship: Corresponding angles are equal.
5. Solve for Unknown Angle: Therefore, the unknown angle also measures 75 degrees.
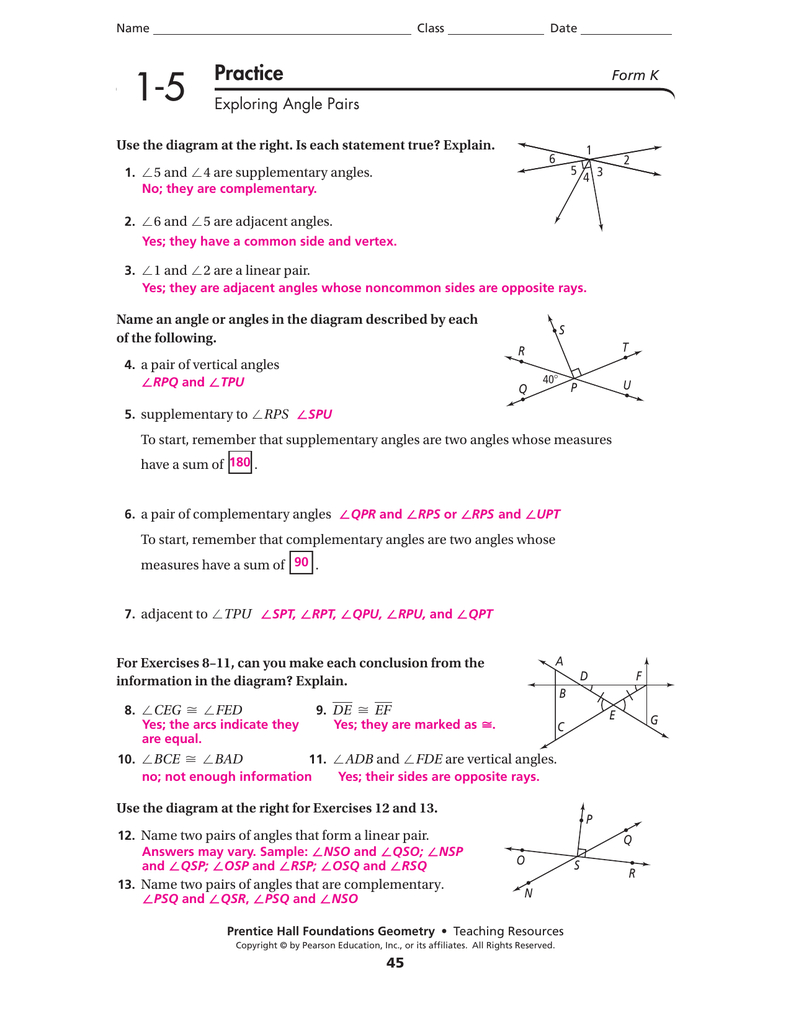
Image: classdbdiederich.z13.web.core.windows.net
Tips and Expert Advice for Tackling Angle Relationships
As you work through angle relationship problems, remember these key tips from seasoned geometry solvers:
1. Draw it out: Always start by drawing a neat and clear diagram of the problem, labelling all the known angles and clearly marking the unknown angles you’re trying to find. A visual representation helps you understand the relationships between angles much better.
2. Highlight Key Information: Highlight the relationships between angles in your diagram using different colors or symbols. This makes it easier for you to visually differentiate between angles that are complementary, supplementary, vertical, or adjacent.
3. Practice, practice, practice: Mastering angle relationships takes practice. Work through as many problems as you can, starting with basic examples and gradually increasing the complexity. The more you solve, the more confident you’ll become.
4. Don’t be afraid to ask for help: If you’re struggling with a specific problem or concept, don’t hesitate to reach out for support. Ask your teacher, a tutor, or a fellow student for clarification.
Frequently Asked Questions (FAQ) about Angle Relationships
Q: What are some real-world applications of angle relationships?
A: Angle relationships are used in many real-world applications, including architecture, engineering, construction, and even art. For example, architects use angle relationships to design buildings and ensure stability, while engineers use them to create bridges and other structures.
Q: How can I find angle relationships in a complex diagram?
A: Break down the complex diagram into smaller, simpler parts. Focus on solving for one angle at a time, building up your understanding of the entire diagram as you go. Use systematic notations and color-coding to differentiate between angles.
Q: What happens when angles aren’t labelled in a diagram?
A: If an angle is not labelled, you can use algebraic notation (such as ‘x’ or ‘y’) to represent its measure. Label it clearly on your diagram and then use the angle relationships to form equations that you can solve for the unknown values.
Using Angle Relationships To Find Angle Measures Answer Key
Conclusion
Understanding angle relationships is a fundamental skill in geometry, unlocking the secrets behind calculating angle measures and solving various geometric problems. By mastering the concepts of complementary, supplementary, vertical, adjacent, corresponding, and alternate angles, you gain a powerful ability to analyze geometrical diagrams and decipher their hidden relationships.
From basic problems to complex diagrams, you can now approach angle measures with confidence, knowing that you hold the key to unlocking their mysteries. So, are you ready to tackle the world of angles with newfound knowledge and skill? Keep practicing, keep exploring, and enjoy the fascinating world of geometry!






